Strategies vs Models
Strategy: How students solve the problem. (Examples: Decomposition, Compensation, Traditional Algorithm)
Model: How students notate their thinking. (Examples: Number line, Ten Frames, Base Ten Blocks)
Important to Know:
One strategy can be explained using multiple models. One model can be used to explain multiple strategies.
Fluency with strategies evolves over time.
The Development of Mathematical Reasoning
Pamela Harris' Development of Mathematical Reasoning: Counting Strategies ⇒ Additive Thinking Strategies ⇒ Multiplicative Reasoning Strategies ⇒ Proportional Reasoning Strategies ⇒ Functional Reasoning Strategies
Students develop mathematical reasoning as they develop more sophisticated thinking. A student who solves 4 x 5 by using counting strategies or additive thinking strategies is not engaging in multiplicative reasoning. Although, this student will correctly determine the answer to 4 x 5 using other strategies, if they do not develop multiplicative reasoning, exploring more complex questions will be extremely challenging. For example, a student may use counting or additive thinking strategies as part of their process for solving 1.2 x 2.3 or (x + 2)(x - 1), but will be unable to solve it without using multiplicative thinking.
When a student develops fluency in multiple strategies and more sophisticated strategies, the student can confidently select the most appropriate strategy based on the numbers.
Naming Strategies:
Mathematical strategies should be named using the math utilized in the strategy. It might be cute to name the strategy after the student who first demonstrates it in class but when a student moves to a different class or a different school, non-conventional naming is not helpful. A grade level team and, preferably, a school, should agree upon names for the strategies that will be explored in math class. There aren't that many of them. Decomposing, compensating, partitioning by place value, give and take, and the traditional algorithm will cover almost all possible strategies.
Strategies for this concept:
Below you will find a short description for each of the most typically used strategies for multiplicative thinking. Each strategy will include one or two models to help notate the thinking within the strategy. When solving a question, a student might use a single strategy or apply two or more strategies. Note that, although the sample question provided below could be solved using other reasoning, only the reasoning appropriate to this concept has been included.
Read about the criteria used to select high leverage strategies.
Examples have been included for each strategy.
Multiplication: 5 x 18
Compensation
Adjust one of the numbers to make it easier to work with while keeping the other number the same. Solve the new question. Adjust the answer to account for (compensate for) the change made to the first number.

Decomposition
Decompose (break apart) one or both of the numbers into more manageable values in order to make it easier to solve.
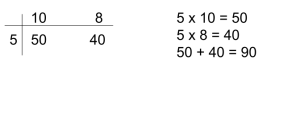
The Distributive Property: Building Arrays and the Area Model: Explore the distributive property through arrays and the area model as it can be developed from Kindergarten to Grade 8.
Multiplication – Decomposition Strategy: These videos explore multiplication through decomposition.
Division- Decomposition Strategy : These videos explore division through decomposition while making connections to the traditional algorithm.
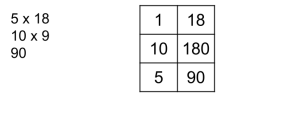
“Doubles / Halving”
The name of this strategy is a misnomer as it is not limited to knowing doubles or halves. You might use triples, thirds, fourths, etc. The first example below focuses on “give and take” where you double the 5 at the same time that you are halving the 18. The second example could relate to compensation as it first doubles the 10, and then halves the result.
Traditional Algorithm
The traditional algorithm (often called the standard algorithm) is a standardized process for solving multiplication and division questions. It focuses on solving a question digit by digit. Caution: The Traditional Algorithm most often described by Canadians is the traditional algorithm for Canada. Other countries have their own traditional algorithms. Keep this in mind if using this phrasing with students. (Source)

Formative Assessment
Ways to show 4 x 3 – 4 questions. Students determine if the example shows a way to think about 4 x 3 and provide an explanation. Student exemplars included. <Original Website>




