Numerate Environment

Making Connections Strengthens Learning

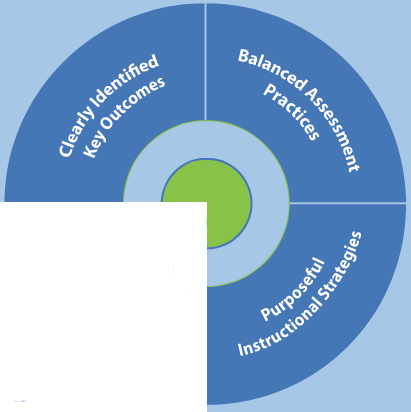
Types of connections

Retrieved from: Gojak, L. , 2013   Project Math: Making Connections, redrawn for web

How do I Support This?

The examples below are taken from the following source: Making Connections in Math (see bibliography section)

CONSIDER

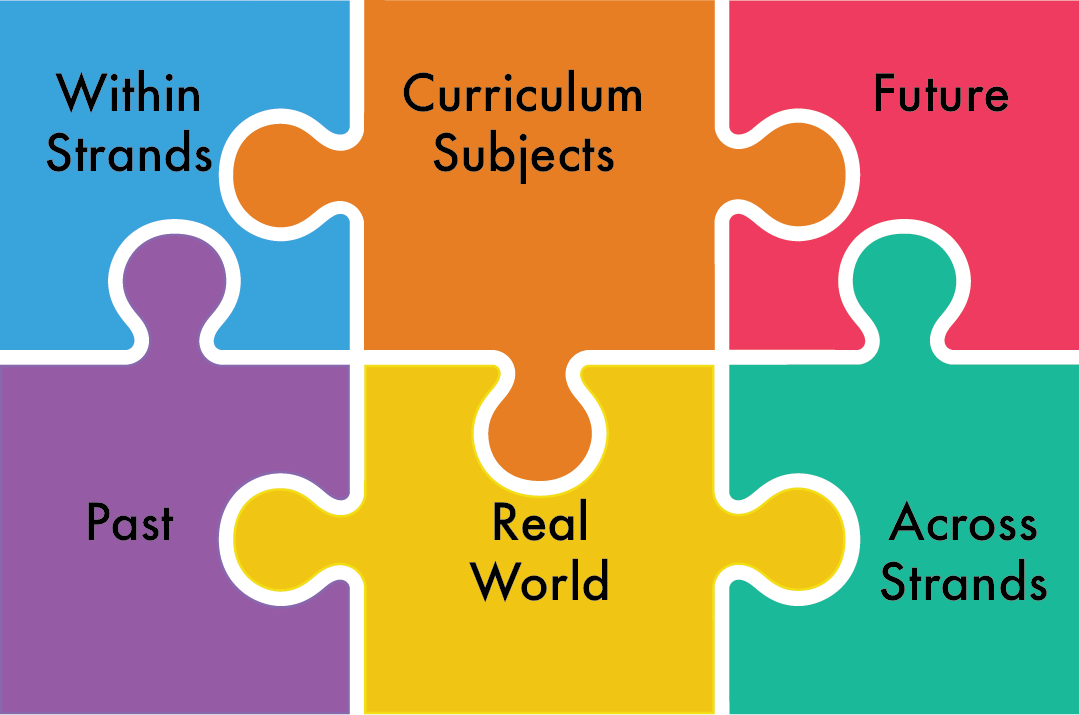
Connections within Strands

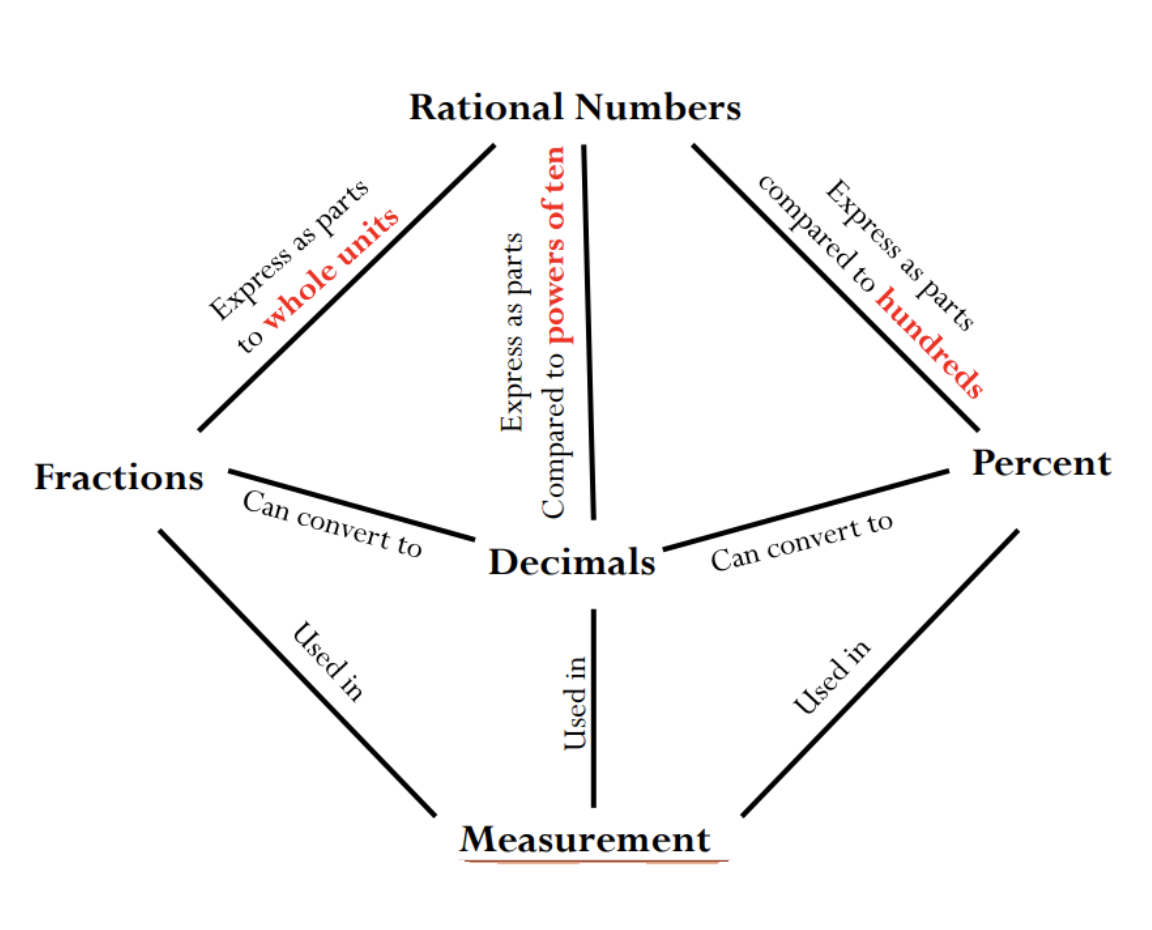
Connecting ideas, concepts or topics within a math strand concretely, pictorially and symbolically (e.g. the connection between rational numbers, percent, decimals, fractions and measurement) (Connections in Math, n.d.).

Connections With Other Curriculum Subjects

• making cross curricular links whenever possible (e.g. to music, science, geography, chemistry, home ec, etc.). 
• Consider having discussions with colleagues in other subject areas to identify and develop agreed upon approaches to the teaching of common procedures in math (Connections in Math, n.d.)

Connections with other Curriculum Subjects (Disciplinary Literacy)

• Meanings of words: explore words that you would find in both Math and English but have different meanings (e.g. Reflection), comparable meanings (e.g. difference), words that are found only in math (e.g. quotient) and words that have more than one math meaning (e.g. round as a circle and round an answer)
• How words are used: explore words that are homonyms with everyday English (e.g. Sum and some), words that are related but students may confuse their meanings (e.g. hundreds and hundredths) and math concepts that are verbalised in different ways (e.g. one quarter versus one fourth)

(Connections in Math, n.d.; Armitage, 2019; Shanahan & Shanahan, 2012)

Future Connections

• Reflecting on the connections among concepts and  across grade levels. How and where does the concept originate, extend and connect with other concepts across the grades?

Past Connections

• Ensure lessons begin with reviewing previous learning.
• Consider connecting current math learning with the history of mathematics – problems that have been present in history, building cultural links and connecting math to society

Real World Connections

• Supporting students in making the connections between “out-of-school” experiences and “in-school-math“

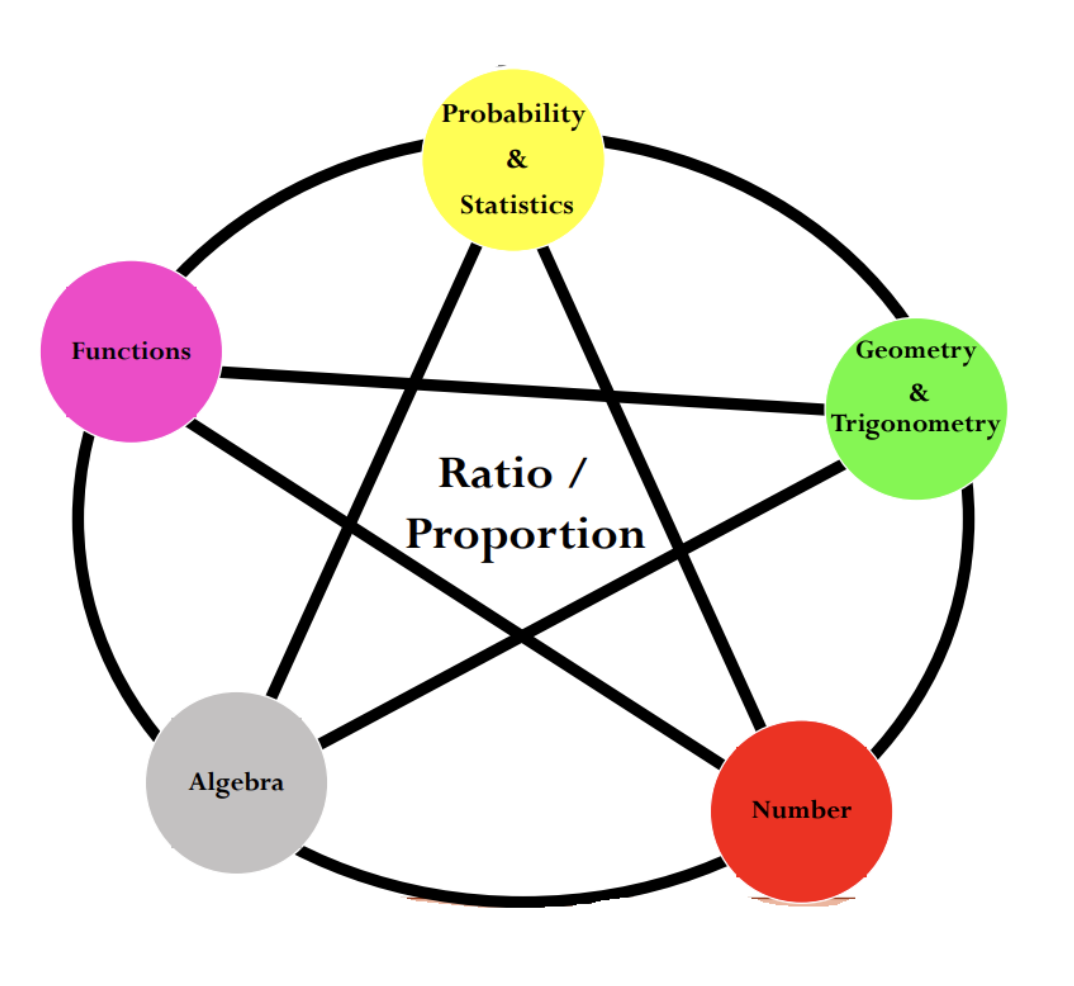
Across Strands Connections

The structure of the program of studies should not be taken as the order in which topics need to be studied in isolation. Whenever possible, connections should be made within and across the strands.

Example:

Instructional Strategies that Help Build Connections

• Encouraging students to represent their thinking in different forms (eg words, a picture, a graph, an equation, and link them).
• Encouraging color coding whereby you ask students to show with color where a mathematical idea is.
• Providing experiences with manipulatives that ask students to make connections between what they are doing with the manipulatives and how that connects with the mathematical concepts that the manipulatives represent (Gojak, 2013).
• Asking students to clarify, explain or  support a part of their solution to a math partner, the whole class, or a teacher, not only helps develop independent problem solvers but also leads to more math connections. By asking students questions that provide them the opportunity to show and share what they know, connections become a natural part of their solutions (Armitage, 2019)

Resources to use with your Class and Students

Children’s Literature

Available in IMC

• Five Creatures by Emily Jenkins
• Seeing Symmetry by Loreen Leedy
• Hat Tricks Count by Matt Napier & Melanie Rose
• A Very Improbable Story by Edward Einhorn
• What’s Your Angle, Pythagoras? By Julie Ellis
• Pythagoras and the Ratios by Julie Ellis
• A Place for Zero by Angeline Sparagna LoPresti

Other Suggested Literature

Cindy Neuschwander books — each book connect to specific math outcomes but also the real world

“Blockhead: The Life of Fibonacci” by Joseph D’Agnese

Stuart J. Murphy books — each book connects to specific math outcomes but also the real world