Place Value

Relating numerals to number of objects

Using simple objects (pennies, tiles, etc.-anything easy to grab per finger)

Dump a pile of about 50 small objects (this example will use 52) in front of you so that all students can see them.

“How many objects do you think there are?” Give students a chance to make a prediction.

Count out the objects, one by one. Using the same finger for each one. Say “I’m worried that I miscounted. How could I count these again but not one-by-one?” Take the 2’s response. Ask one student to come stand on your right to help you count. That student will be keeping track out loud of the number of times you’ve counted a pile of 2. (ie. one 2; two 2s; three 2s; etc.) Ask a student to come stand on your left to help you count. That student will be keeping track out loud of the current count. (ie. 2, 4, 6). The first time you do this, you will probably have to help prompt the first student until they understand the format for counting. Any time you grab items, you will grab one per finger. Make it obvious! Grab 2. Student 1 will say “one 2”. Student 2 will say “2”. Grab two more. Student 1 will say “two 2s”. Student 2 will say “4”.

Ask if there’s another way to count them, other than by 1s or 2s. Take the 5’s response. Repeat the process of counting again. Make sure that each finger on one hand pulls an object towards you when counting. Student 1 says 1 five, 2 fives, 3 fives, 4 fives, 5 fives, 6 fives, 7 fives, 8 fives, 9 fives, 10 fives, 10 fives and 1, 10 fives and 2. Student 2 says 5, 10, 15. 20, 25, 30, 35, 40, 45, 50, 51, 52

Ask again. Take the 10”s answer. Repeat the process, using both hands – one object per finger.

Sweep the pennies to the side. Say, “Yesterday, I was counting out a different pile of pennies. I counted 2 tens and 3. How many pennies did I have?” Give students time to work through it and talk with a partner. You have model this using Student 1 and 2. 

Record 23 on the board. Point to the 3 and ask “Does this part of the twenty-three have anything to do with the pennies we counted?” Point to the 2 and ask “Does this part of the twenty-three have anything to do with the pennies we counted?”

Record 41 on the board. Ask them to discuss each of the digits and relate them to the activity you just did.

Using simple objects (tiles, unifix cubes, etc. but NOT base ten blocks) (Ross, 1989)

If you are working one-on-one with a student, place 25 objects in front of the student. If working as a large group, display 25 objects on the board and provide students with the same image on paper.

Ask, “How many are there?” Give the student(s) time to figure it out. (Ensure that the class comes to a correct consensus.) Have the student(s) recheck if incorrect.

Have the student(s) write down the answer on the paper.

Circle the 5 on the board. “Does this part of your twenty-five have anything to do with how many objects you have/see?” The class can use pencil crayons to show. (Do NOT say the number 5 as it will influence students’ thinking)

Circle the 2. “Does this part of your twenty-five have anything to do with how many objects you have/see?” The class can show using their pencil crayons. (Do NOT say the number 2 as it will influence students’ thinking)

Do students understand that the 5 represents 5 objects and the 2 represents 20? 

Using base ten blocks (Ross, 1989)

Provide  display 52 using 4 tens and 12 ones. Repeat the process above.

Provide / display 52 using 5 tens and 2 ones. Repeat the process above.

Conservation of Number as it relates to place value:

What is “Conservation of Number?” Conservation of Number is the idea that the number of objects remains the same even when rearranged.

Misconception: Students may believe that exchanging base ten blocks increases or decreases the value of the number.

The importance of this concept:  Students who do not understand conservation of number will struggle with place value and operations.  Imagine a student using base ten blocks to subtract. When they have to exchange one ten for ten ones, they will have to recount the blocks each time. When adding 4 + 3, they may need to “count all”: 1-2-3-4…5-6-7

At what grade level is this appropriate to begin? The concept of place value appears in the grade 2 curriculum. 

How to address the Misconception: 

Activity 1:

Ensure that students have spent time working with base ten blocks before trying this activity.

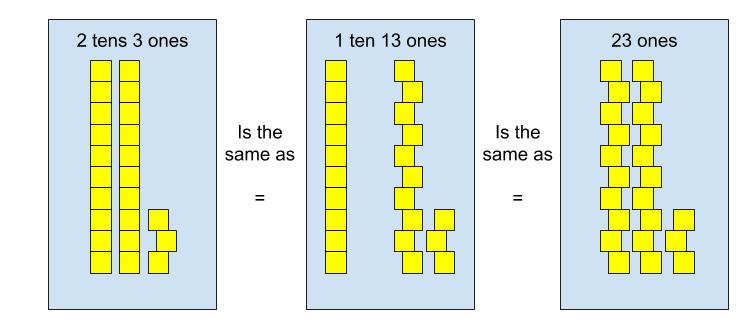
Ask students to represent 23 using base ten blocks. (Ensure they are not “drawing” the number 23. This happens sometimes.)

As students are building, walk around the classroom to see how many different ways are being represented. Watch for students building it using 23 ones, 2 tens 3 ones, and 1 ten 13 ones.

As students finish, ask them individually, “Can you find another way to represent 23?”

Only give a few minutes for this task.

Ask the class, “Who would like to share how they represented 23?” Choose a student who represented it using 2 tens 3 ones.

“Who else represented it using 2 tens 3 ones?”

“Who represented it a different way?” Choose a student who used 23 ones.

“Who else represented it using 23 ones?”

“Did anyone represent it a different way?” Don’t let the student give their response.

“Interesting. I wonder how they represented it differently. I’ll give you two minutes to see if you can find a third way to represent this number.”

After a few minutes, discuss the third representation of 1 ten and 13 ones.

Ask, “We just saw three different ways of representing 23. Which one is correct?”

“They all are! Each of the three representations are useful. You will need to figure out which one is most helpful in the situation you are working with.”

Let students choose other numbers. How many different ways can you represent your number?

This activity can be repeated using decimal numbers.

Activity 2:

Display 34 on the board using base ten blocks – 3 tens and 4 ones.

“What is this number?”

“If I trade / exchange one ten for ten ones, will my new number be larger than, smaller than, or the same as 34? Take a moment to think about it by yourself. Discuss with your partner.”

“Who would like to share either their thinking or their seat partner’s thinking?”

This activity can be repeated using decimal numbers.